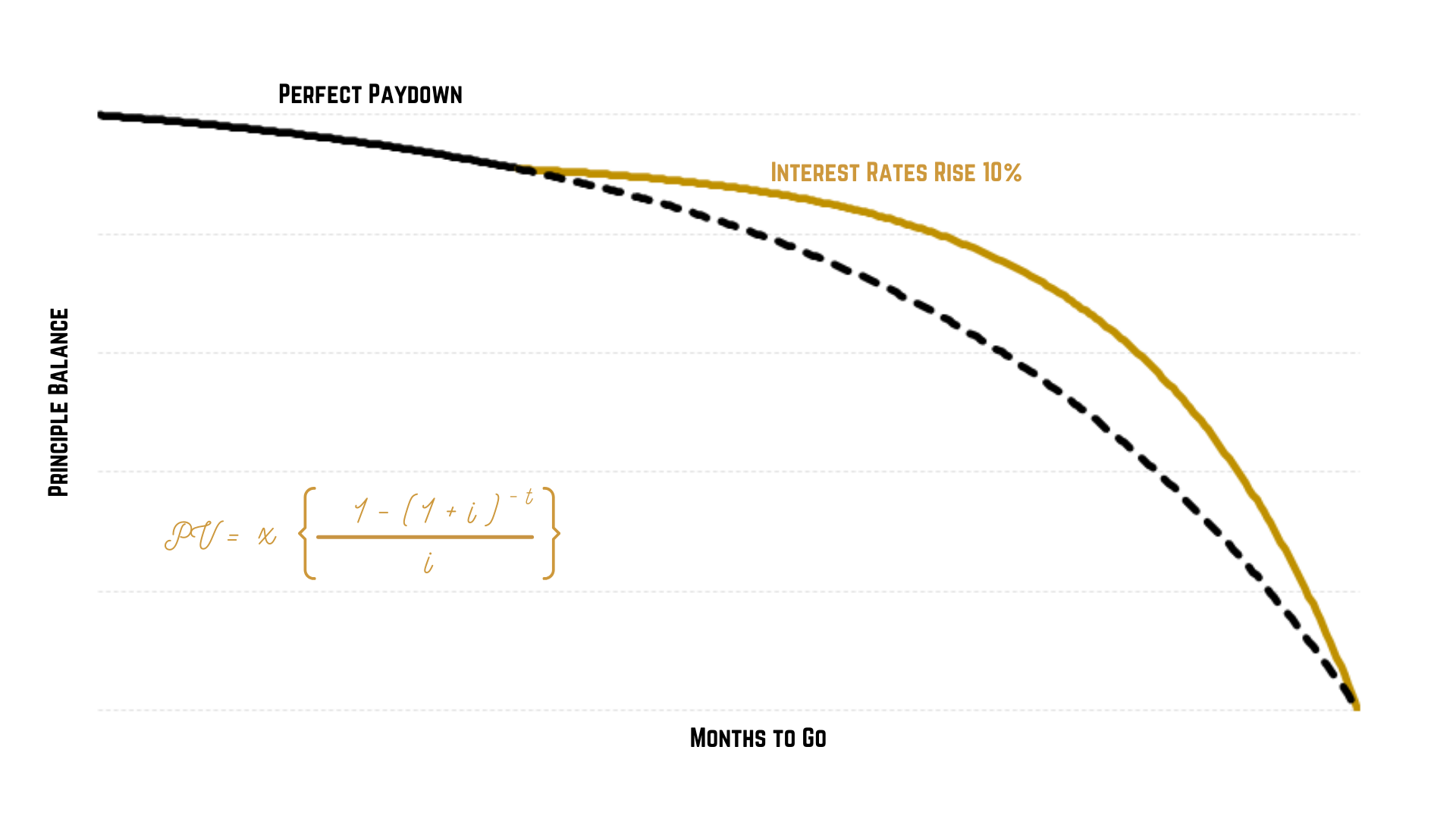
Perfect paydown curves
PV = x [ (1 – (1 + i) -t )/ i ]
Where:
PV = the present value of the loan at a given point in time
x = the instalment amount
i = the interest rate
t = the number of terms remaining
You can shuffle this all around as needed, so that: if the PV is lower than the actual balance outstanding, a customer is in arrears (in the old days I had to do this sometimes when we took on portfolios with questionable data hygiene but that’s seldom needed these days); if in collections, you need to reduce the instalment by a certain amount, you can see how many months the loan would be extended by; or, in the affordability context; if you have calculated the maximum monthly instalment and have a set term and price, what the largest affordable loan is.

Risk-based pricing
This situation is doubly problematic as it doesn’t only lead to sub-optimal profit but also to a sub-optimal portfolio structure. The lowest risk customers (who are being over-charged) can be tempted to leave for cheaper competitor offers while the higher risk customers (who are being under-charged) will gravitate towards your product; leading to a riskier portfolio on average. Risk-based pricing addresses this by lowering the rates charged to low-risk customers and raising the rates charged to high-risk customers.
The key to successful risk-based pricing is, not surprisingly, a good understanding of risk so scorecards are once again at the heart of any strategy. But now we’re not thinking in terms of a binary approve/ decline decision. Instead, we’re thinking on a near-continuous spectrum where we accept everyone at the right price (only declining when the ‘right price’ is too high to be legally, ethically, or otherwise acceptable).

