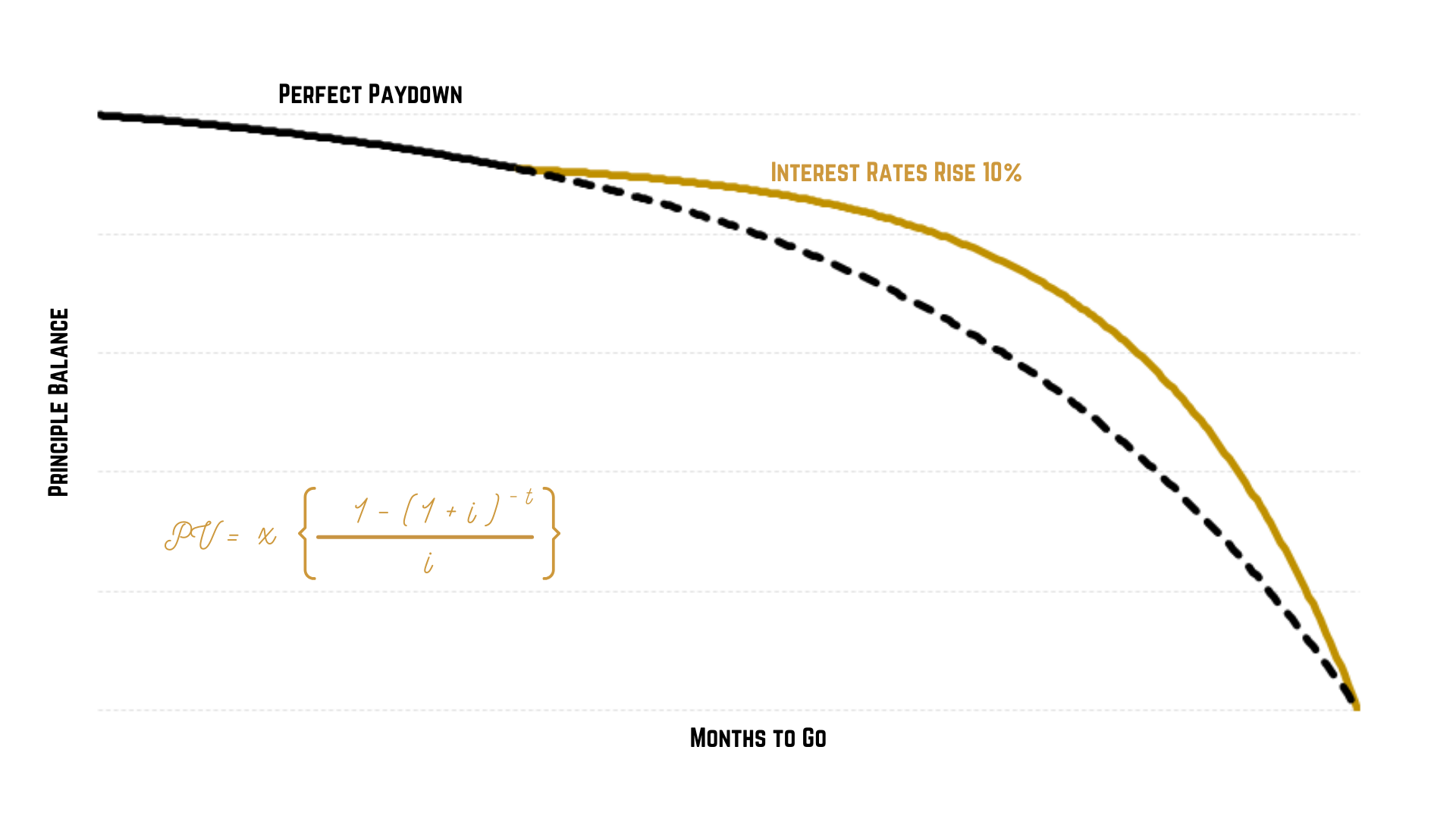
Perfect paydown curves
PV = x [ (1 – (1 + i) -t )/ i ]
Where:
PV = the present value of the loan at a given point in time
x = the instalment amount
i = the interest rate
t = the number of terms remaining
You can shuffle this all around as needed, so that: if the PV is lower than the actual balance outstanding, a customer is in arrears (in the old days I had to do this sometimes when we took on portfolios with questionable data hygiene but that’s seldom needed these days); if in collections, you need to reduce the instalment by a certain amount, you can see how many months the loan would be extended by; or, in the affordability context; if you have calculated the maximum monthly instalment and have a set term and price, what the largest affordable loan is.

Affordability versus Risk
A good credit history and money management skills speak to a consumer’s willingness to repay, affordability checks speak to their ability to repay. And, at least in theory, calculating a consumer’s ability to repay a loan should be the simpler of the two tasks, being that ‘ability’ is all about the numbers while ‘willingness’ requires us to get inside the borrower’s head to some extent. Of course, it is not so easy in the real world, but simply put, a consumer is able to repay a loan when they have more money available to meet their debt obligations than they need to keep those obligations up-to-date.

